Verifiable secret sharing: Difference between revisions
en>ChrisGualtieri m →Feldman’s scheme: TypoScan Project, typos fixed: , → , using AWB |
en>Widefox Added {{no footnotes}} tag to article (TW) |
||
| Line 1: | Line 1: | ||
In [[topology]] and in [[calculus]], a '''round function''' is a [[scalar function]] <math>M\to{\mathbb{R}}</math>, | |||
over a [[manifold]] <math>M</math>, whose [[critical point (mathematics)|critical point]]s form one or several [[connected space|connected component]]s, each [[homeomorphic]] to the [[circle]] | |||
<math>S^1</math>, also called critical loops. They are special cases of [[Morse-Bott function]]s. | |||
[[Image:Critical-loop.PNG|right|thumb|300px|The black circle in one of this critical loops.]] | |||
==For instance== | |||
For example, let <math>M</math> be the [[torus (mathematics)|torus]]. Let | |||
:<math>K=(0,2\pi)\times(0,2\pi).\,</math> | |||
Then we know that a map | |||
:<math>X\colon K\to{\mathbb{R}}^3\,</math> | |||
given by | |||
:<math>X(\theta,\phi)=((2+\cos\theta)\cos\phi,(2+\cos\theta)\sin\phi,\sin\theta)\,</math> | |||
is a parametrization for almost all of <math>M</math>. Now, via the projection <math>\pi_3\colon{\mathbb{R}}^3\to{\mathbb{R}}</math> | |||
we get the restriction | |||
:<math>G=\pi_3|_M\colon M\to{\mathbb{R}}, (\theta,\phi) \mapsto \sin \theta \,</math> | |||
<math>G=G(\theta,\phi)=\sin\theta</math> is a function whose critical sets are determined by | |||
:<math>\nabla G(\theta,\phi)= | |||
\left({{\partial}G\over {\partial}\theta},{{\partial}G\over {\partial}\phi}\right)\!\left(\theta,\phi\right)=(0,0),\,</math> | |||
this is if and only if <math>\theta={\pi\over 2},\ {3\pi\over 2}</math>. | |||
These two values for <math>\theta</math> give the critical sets | |||
:<math>X({\pi/2},\phi)=(2\cos\phi,2\sin\phi,1)\,</math> | |||
:<math>X({3\pi/2},\phi)=(2\cos\phi,2\sin\phi,-1)\,</math> | |||
which represent two extremal circles over the torus <math>M</math>. | |||
Observe that the [[Hessian matrix|Hessian]] for this function is | |||
:<math>{\rm Hess}(G)= | |||
\begin{bmatrix} | |||
-\sin\theta & 0 \\ 0 & 0 \end{bmatrix} | |||
</math> | |||
which clearly it reveals itself as of <math>{\rm rank Hess}(G)=1</math> | |||
at the tagged circles, making the critical point degenerate, that is, showing that the critical points are not isolated. | |||
==Round complexity== | |||
Mimicking the [[Lyusternik-Schnirelmann category|L-S]] category theory one can define the '''round complexity''' asking for whether or not exist round functions on manifolds and/or for the minimum number of critical loops. | |||
==References== | |||
* Siersma and Khimshiasvili, ''On minimal round functions'', Preprint 1118, Department of Mathematics, Utrecht University, 1999, pp. 18.[http://citeseer.ist.psu.edu/287481.html]. An update at [http://igitur-archive.library.uu.nl/math/2001-0628-161022/1118.pdf] | |||
[[Category:Differential geometry]] | |||
[[Category:Geometric topology]] | |||
[[Category:Types of functions]] | |||
Latest revision as of 15:49, 20 July 2013
In topology and in calculus, a round function is a scalar function , over a manifold , whose critical points form one or several connected components, each homeomorphic to the circle , also called critical loops. They are special cases of Morse-Bott functions.
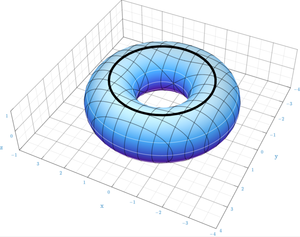
For instance
For example, let be the torus. Let
Then we know that a map
given by
is a parametrization for almost all of . Now, via the projection we get the restriction
is a function whose critical sets are determined by
These two values for give the critical sets
which represent two extremal circles over the torus .
Observe that the Hessian for this function is
which clearly it reveals itself as of at the tagged circles, making the critical point degenerate, that is, showing that the critical points are not isolated.
Round complexity
Mimicking the L-S category theory one can define the round complexity asking for whether or not exist round functions on manifolds and/or for the minimum number of critical loops.















