Regular category: Difference between revisions
en>LokiClock "Kernel pair" is here |
Deltahedron (talk | contribs) →Exact (effective) categories: cite Pedicchio & Tholen (2004) |
||
| Line 1: | Line 1: | ||
In [[mathematics]], the '''no-wandering-domain theorem''' is a result on [[dynamical system]]s, proven by [[Dennis Sullivan]] in 1985. | |||
The theorem states that a [[rational function|rational map]] ''f'' : '''Ĉ''' → '''Ĉ''' with deg(''f'') ≥ 2 does not have a [[wandering domain]], where '''Ĉ''' denotes the [[Riemann sphere]]. More precisely, for every [[Connected space|component]] ''U'' in the [[Fatou set]] of ''f'', the sequence | |||
:<math>U,f(U),f(f(U)),\dots,f^n(U), \dots</math> | |||
will eventually become periodic. Here, ''f''<sup> ''n''</sup> denotes the [[function iteration|''n''-fold iteration]] of ''f'', that is, | |||
:<math>f^n = \underbrace{f \circ f\circ \cdots \circ f}_n .</math> | |||
[[File:Wandering_domains_for_the_entire_function_f%28z%29%3Dz%2B2πsin%28z%29.png|thumb|alt=An image of the dynamical plane for <math>f(z)=z+2\pi\sin(z)</math>.|This image illustrates the dynamics of <math>f(z)=z+2\pi\sin(z)</math>; the Fatou set (consisting entirely of wandering domains) is shown in white, while the Julia set is shown in tones of gray.]] | |||
The theorem does not hold for arbitrary maps; for example, the [[Transcendental function|transcendental map]] <math>f(z)=z+2\pi\sin(z)</math> has wandering domains. However, the result can be generalized to many situations where the functions naturally belong to a finite-dimensional parameter space, most notably to transcendental entire and meromorphic functions with a finite number of singular values. | |||
==References== | |||
* [[Lennart Carleson]] and Theodore W. Gamelin, ''Complex Dynamics'', Universitext: Tracts in Mathematics, [[Springer-Verlag]], New York, 1993, ISBN 0-387-97942-5 {{MathSciNet| id=1230383}} | |||
* Dennis Sullivan, ''Quasiconformal homeomorphisms and dynamics. I. Solution of the Fatou-Julia problem on wandering domains'', [[Annals of Mathematics]] 122 (1985), no. 3, 401–18. {{MathSciNet| id=0819553}} | |||
* S. Zakeri, ''[http://www.math.qc.edu/~zakeri/notes/wander.pdf Sullivan's proof of Fatou's no wandering domain conjecture]'' | |||
{{mathapplied-stub}} | |||
[[Category:Ergodic theory]] | |||
[[Category:Limit sets]] | |||
[[Category:Theorems in dynamical systems]] | |||
[[Category:Complex dynamics]] | |||
Revision as of 19:50, 29 March 2013
In mathematics, the no-wandering-domain theorem is a result on dynamical systems, proven by Dennis Sullivan in 1985.
The theorem states that a rational map f : Ĉ → Ĉ with deg(f) ≥ 2 does not have a wandering domain, where Ĉ denotes the Riemann sphere. More precisely, for every component U in the Fatou set of f, the sequence
will eventually become periodic. Here, f n denotes the n-fold iteration of f, that is,
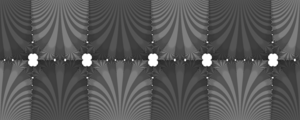
The theorem does not hold for arbitrary maps; for example, the transcendental map has wandering domains. However, the result can be generalized to many situations where the functions naturally belong to a finite-dimensional parameter space, most notably to transcendental entire and meromorphic functions with a finite number of singular values.
References
- Lennart Carleson and Theodore W. Gamelin, Complex Dynamics, Universitext: Tracts in Mathematics, Springer-Verlag, New York, 1993, ISBN 0-387-97942-5 Template:MathSciNet
- Dennis Sullivan, Quasiconformal homeomorphisms and dynamics. I. Solution of the Fatou-Julia problem on wandering domains, Annals of Mathematics 122 (1985), no. 3, 401–18. Template:MathSciNet
- S. Zakeri, Sullivan's proof of Fatou's no wandering domain conjecture


